Pajek/GlossGT
Pajek network: graph and digraph glossary
| Name |
GlossGT |
| Group |
Pajek |
| Matrix ID |
1501 |
|
Num Rows
|
72 |
|
Num Cols
|
72 |
|
Nonzeros
|
122 |
|
Pattern Entries
|
122 |
|
Kind
|
Directed Graph |
|
Symmetric
|
No |
|
Date
|
2001 |
|
Author
|
W. Cherowitzo |
|
Editor
|
V. Batagelj |
| Structural Rank |
|
| Structural Rank Full |
|
|
Num Dmperm Blocks
|
|
|
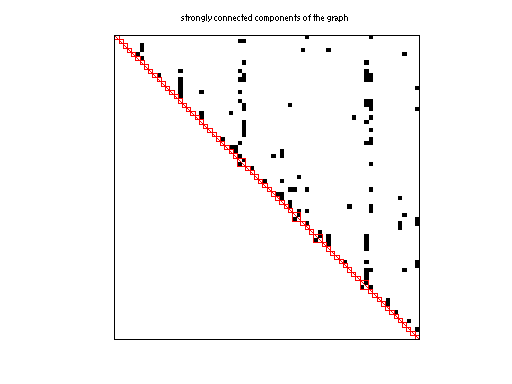
Strongly Connect Components
|
68 |
|
Num Explicit Zeros
|
0 |
|
Pattern Symmetry
|
6.6% |
|
Numeric Symmetry
|
6.6% |
|
Cholesky Candidate
|
no |
|
Positive Definite
|
no |
|
Type
|
binary |
| SVD Statistics |
| Matrix Norm |
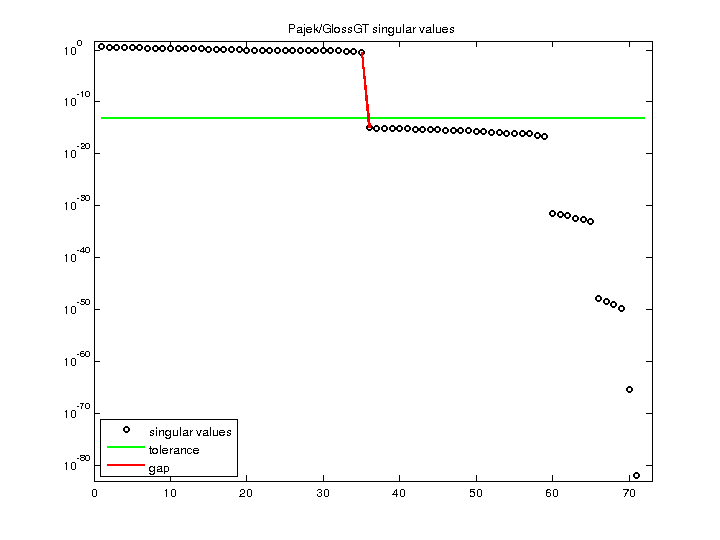
5.406244e+00 |
| Minimum Singular Value |
0 |
| Condition Number |
Inf
|
| Rank |
35 |
| sprank(A)-rank(A) |
|
| Null Space Dimension |
37 |
| Full Numerical Rank? |
no |
| Download Singular Values |
MATLAB
|
| Download |
MATLAB
Rutherford Boeing
Matrix Market
|
| Notes |
------------------------------------------------------------------------------
Pajek network converted to sparse adjacency matrix for inclusion in UF sparse
matrix collection, Tim Davis. For Pajek datasets, See V. Batagelj & A. Mrvar,
http://vlado.fmf.uni-lj.si/pub/networks/data/.
------------------------------------------------------------------------------
Bill Cherowitzo: Graph and Digraph Glossary
http://www-math.cudenver.edu/~wcherowi/courses/m4408/glossary.html
Pajek's network: Barbara Zemlji"c, 2. nov 2003
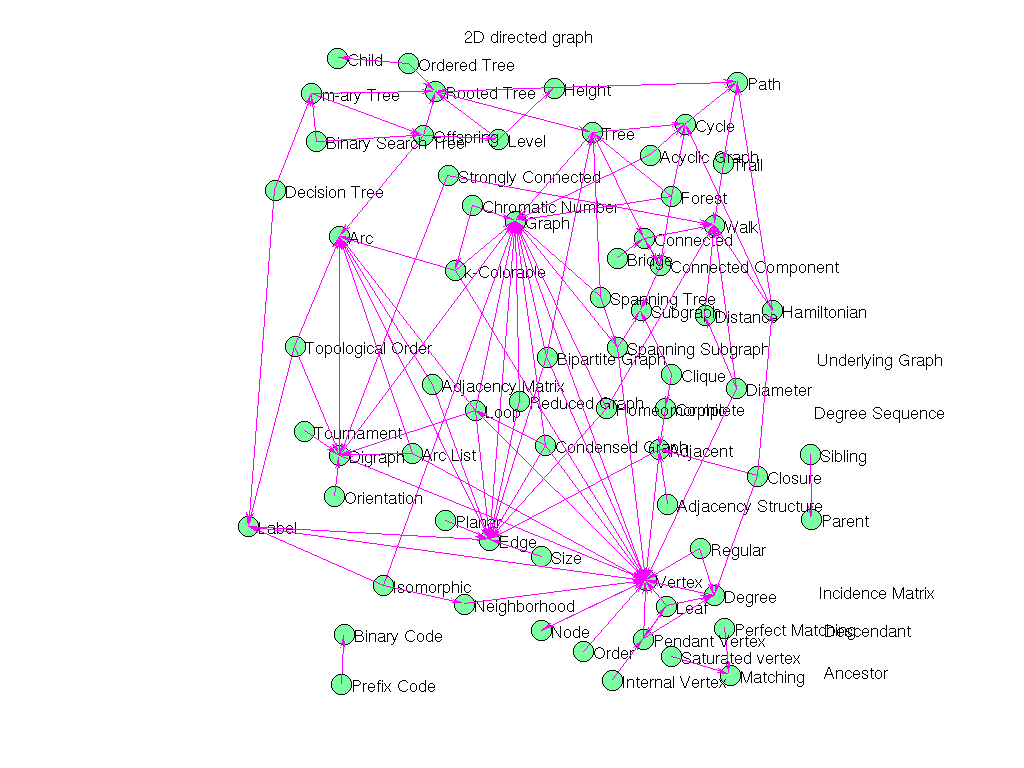
The original problem had 3D xyz coordinates, but all values of z were equal
to 0, and have been removed. This graph has 2D coordinates.
|